Using the same argument as previously, it can be shown that the reconstructed density produced by a gradient-echo acquisition (ge_seq) can be written:
where 
is the echo time. Comparing with
rec_im, this shows clearly that gradient-echo, as
opposed to spin-echo images, contain a ``natural'' phase factor. In
other words, gradient-echo acquisition does not remove the effect of
field inhomogeneities.
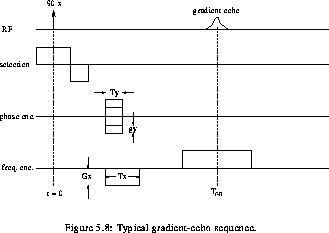
Again, consider three acquisitions (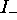
,
,
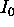) with echo time
of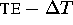 
, 
and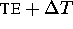 
respectively. Choosing 
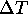so that 
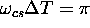, the three acquisitions are given by:
where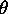 
is a phase shift common to all acquisitions.
It can be shown that the water and fat images are
given by:
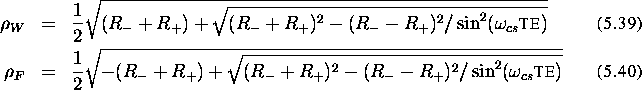
Note that these equations break down when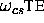 
is an integer multiple of 
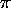. In these situations however,
ge- to ge+ reduce to se- to se+
with the sign of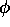 
reversed and with an extra constant phase
factor that can be included in 
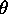. So, one can always choose the
echo-time of a gradient-echo sequence so that the simpler equations of
the spin-echo case can be used to compute the water and fat images.
